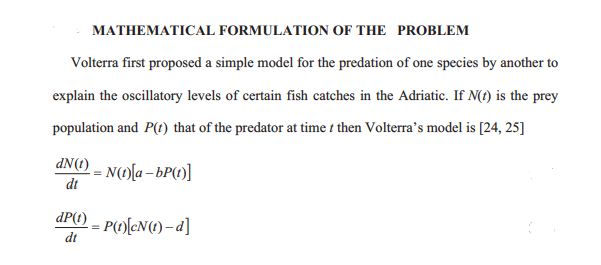
Lotka-volterra predator-pey equations model

Mathematical modeling will always be an important field of mathematics because of its applications to the real world. While no model is perfet, if a close enough approximation can be obtained, then scientists can see how certain factors will affect a situation by merely working out equations on a piece of paper as opposed to actually running an experiment. One mathematical model that is frequently examined is the Lotka-Volterra predator-prey model. This refers to a system in which there are two populations known as the predator and the prey. The model states that the prey will grow at a certain rate but will also be eaten at a certain rate because of predators. The predators will die at a certain rate but will then grow by eating prey. In the recent decades, considerable work on the permanence, the extinction and the global asymptotic stability of autonomous or nonautonomous Lotka-Volterra type predator–prey systems have been studied extensively, for example . In addition to these, the book by Takeuchi [2010] is a good source for dynamical behavior of LotkaVolterra systems. The predator–prey problem attempts to model the relationship in the populations of different species that share the same environment where some of the species (predators) prey on the others. The prey is assumed to exhibit linear growth given by a positive parameter. Predator species consume preys with a nonlinear interaction with another set of parameters that determine the rate of competition between predators. The natural death rate of the predator is assumed to be linear and given by a negative parameter. One of the earliest implementations, the Lotka–Volterra model serves as a starting point of more advanced models in the analysis of population dynamics. Because of its unrealistic stability characteristics, stability analysis of the model and its generalizations has recently gained much attention.

BUY FOR 15,000